In the case of transition from the spontaneous radiation mode to the
stimulated mode, the number of axions (the number of quanta) per an axion
radiation oscillator
n
a
approaches to the number of quanta per an oscillator
of the exciting electromagnetic radiation
n
γ
. If the conditions for transition
to the stimulated radiation are also satisfied in the reconversion process,
the intensity of light radiation at the second cavity output must be large
enough to be registered by the modern detectors.
Another opportunity for the efficient increase of photon-axion and
axion-photon conversion processes is the use of an extended material
medium, placed in an external magnetic field, instead of cavities (vacuum
resonators). Here the problem of satisfying the conditions for synchronism
occurs (4), (5). This problem can be solved by adjusting the photon
frequency participating in the photon-axion conversion process to the
frequency of the so-called unitary polaritons of the material medium,
for which the refractive index module is close to one. A very promising
material for the solution are ruby crystals, an active lasing medium. With
account taken of the three resonance transitions in chromium ions, the law
of polariton dispersion in this crystal can be represented as follows:
ω
2
=
A
2
0
k
2
ε
(
ω
)
,
where
с
0
is the velocity of light in a free space;
ε
(
ω
) =
ε
∞
j
=3
Y
j
−
1
(
ω
2
lj
−
ω
2
)
(
ω
2
tj
−
ω
2
)
.
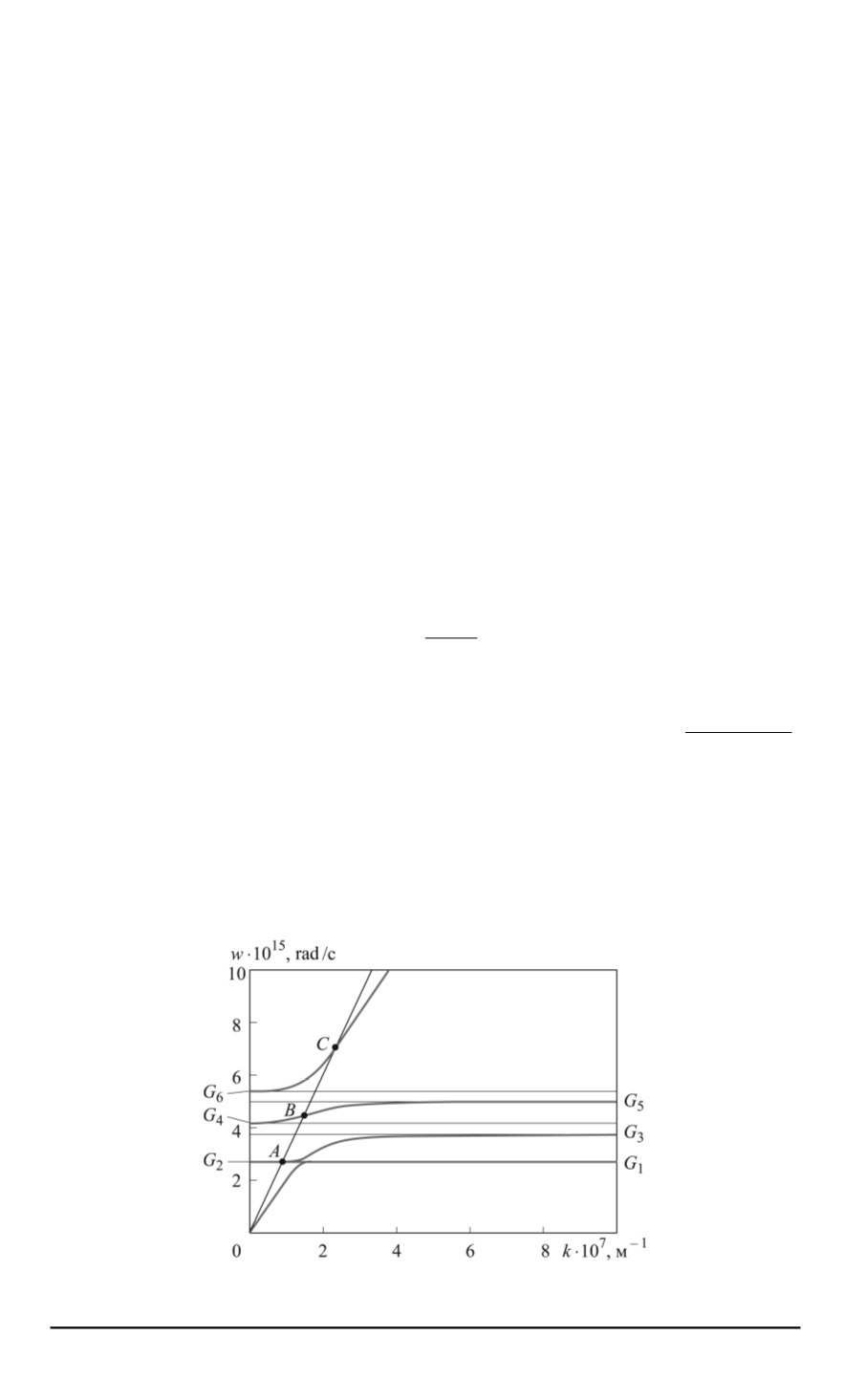
The view of the polariton curves in ruby crystals is shown in Fig. 3. The
points
G
1
, G
3
and
G
5
are related to frequences
ω
tj
of the transverse modes,
while points
G
2
, G
4
and
G
6
are related to frequences
ω
lj
of longitudinal
modes, and points
А
−
С
— to unitary polaritons (
|
n
= 1
|
). In ruby crystals
there occurs a laser generation (
λ
= 694
.
3
nm) of giant (100MW/cm
2
)
Fig. 3. View of the polariton curves in a ruby monocrystal (
Al
2
O
3
: Cr
3
+
)
ISSN 1812-3368. Herald of the BMSTU. Series “Natural Sciences”. 2014. No. 6
11