
The Research of Solution of Levinson — Smith Equation
ISSN 1812-3368. Вестник МГТУ им. Н.Э. Баумана. Сер. Естественные науки. 2017. № 1
19
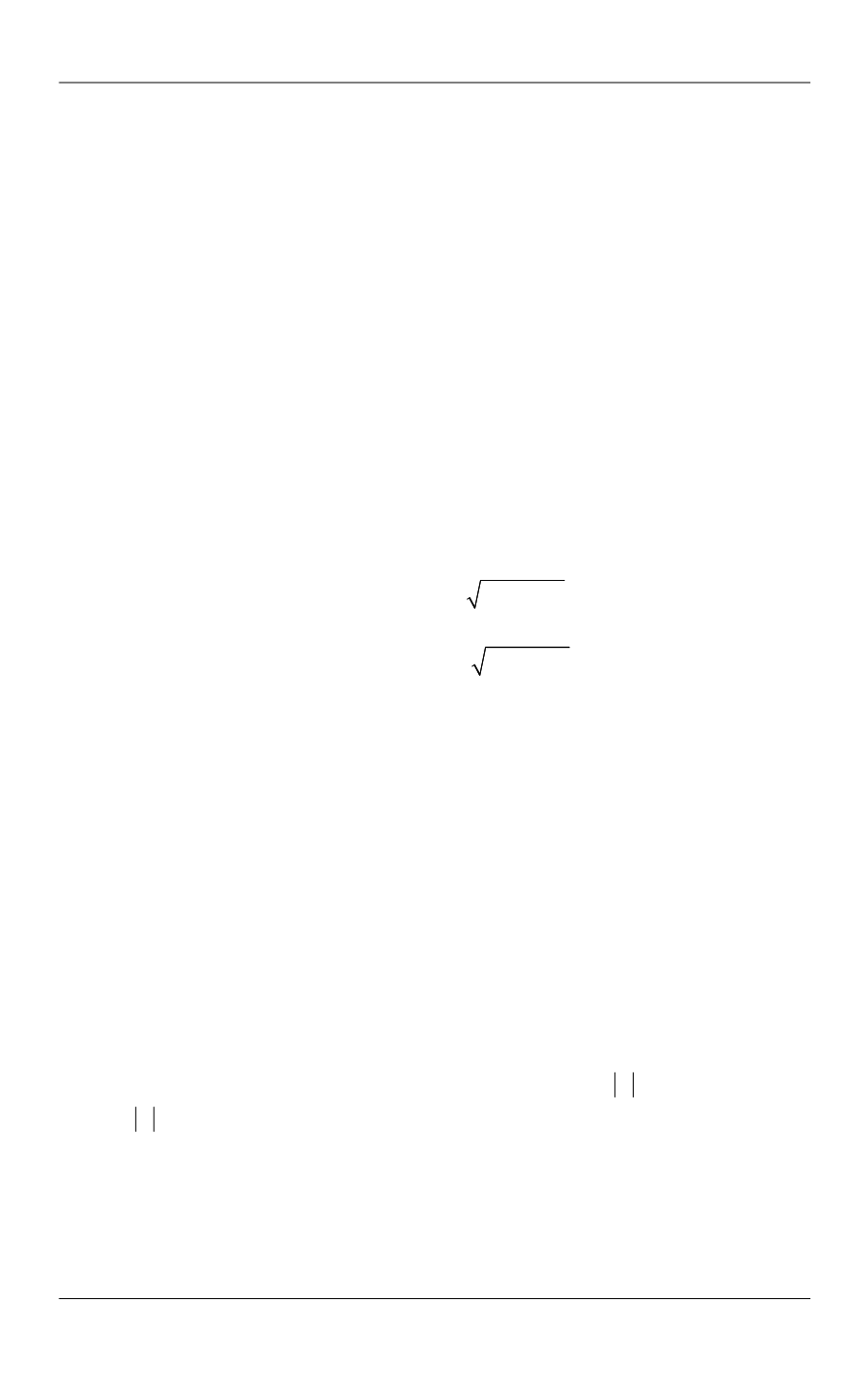
Corollary 1, the set
\
is positively invariant, and, therefore, so are all of its
connected components
,
I
.
I
From above, we conclude the following.
Statement 2.
All the sets
,
I
,
I
are positively invariant.
For an arbitrary
> 0
C
, denote by
( )
U C
the subset defined by the inequalities
> 0
x
and
( )< < ( )
x y x C
and by
( )
U C
the subset defined by the inequalities < 0
x
and
( ) < < ( ).
x C y x
The following theorem is the main result of the paper.
Theorem 1.
Suppose that the system
(5)
satisfies
>0 inf{ ( ) :
( )}> 0,
inf{ ( ) :
( )}>0.
C
f p p U C
f p p U C
(6)
If
:
( ( ), ( )),
t x t y t
0,
t
is an arbitrary trajectory, then
enters the localizing set
at some time
0
0
t
and then never leaves it, or
is completely located outside the
localizing set
,
*
( ( ))
,
t
h t
G
dist( ( ), )
0
t
t
(dist( ,
)
P M
is the
distance from the point P to the set M) and one of the following three conditions holds
1)
( )
;
t
t
p
2)
( )
t
x t
and the integral
*
0
( )
G G x dx
is convergent;
3)
( )
t
x t
and the integral
0
*
( )
G G x dx
is convergent.
◄
If the trajectory enters the localizing set
at some time
0
0,
t
then it never
leaves it since the set
is positively invariant.
Suppose that
is completely located outside the localizing set
.
This means
that
is contained in the set
U
and, therefore, in one of its connected components
U
and
.
U
Then, there are two possible cases
1)
U
and, thus,
= > ( ) 0,
x y x
on
,
i. e.
( )
x t
is strictly increasing and,
hence,
*
( )
( ;
];
t
x t
x
2)
U
and, thus,
= < ( ) 0
x y
x
on
,
i. e.
( )
x t
is strictly decreasing
and, hence,
*
( )
[ ;
).
t
x t
x
In
both
cases,
the
function
( )
x t
is
strictly
monotonous,
*
( )
[ ;
],
t
x t
x
and the function
( )
y t
is nonzero and of constant sign
on
.
The set
is defined by each of two equivalent inequalities
( )
y x
and
*
.
h G
Hence,
> ( )
y x
and
*
>
h G
on
.
By Statement 1, the function
h
is decreasing
on
.
Thus,
*
*
( ( ))
[ ;
)
t
h t
h G
and
*
h h
on
.
Lemma 1.
If
is completely located outside the localizing set
and
*
x
is a finite
number, then the trajectory
tends to some point
p
and, also,
dist( ( ), )
0,
t
t
*
*
( ( ))
= .
t
h t
h G
















