
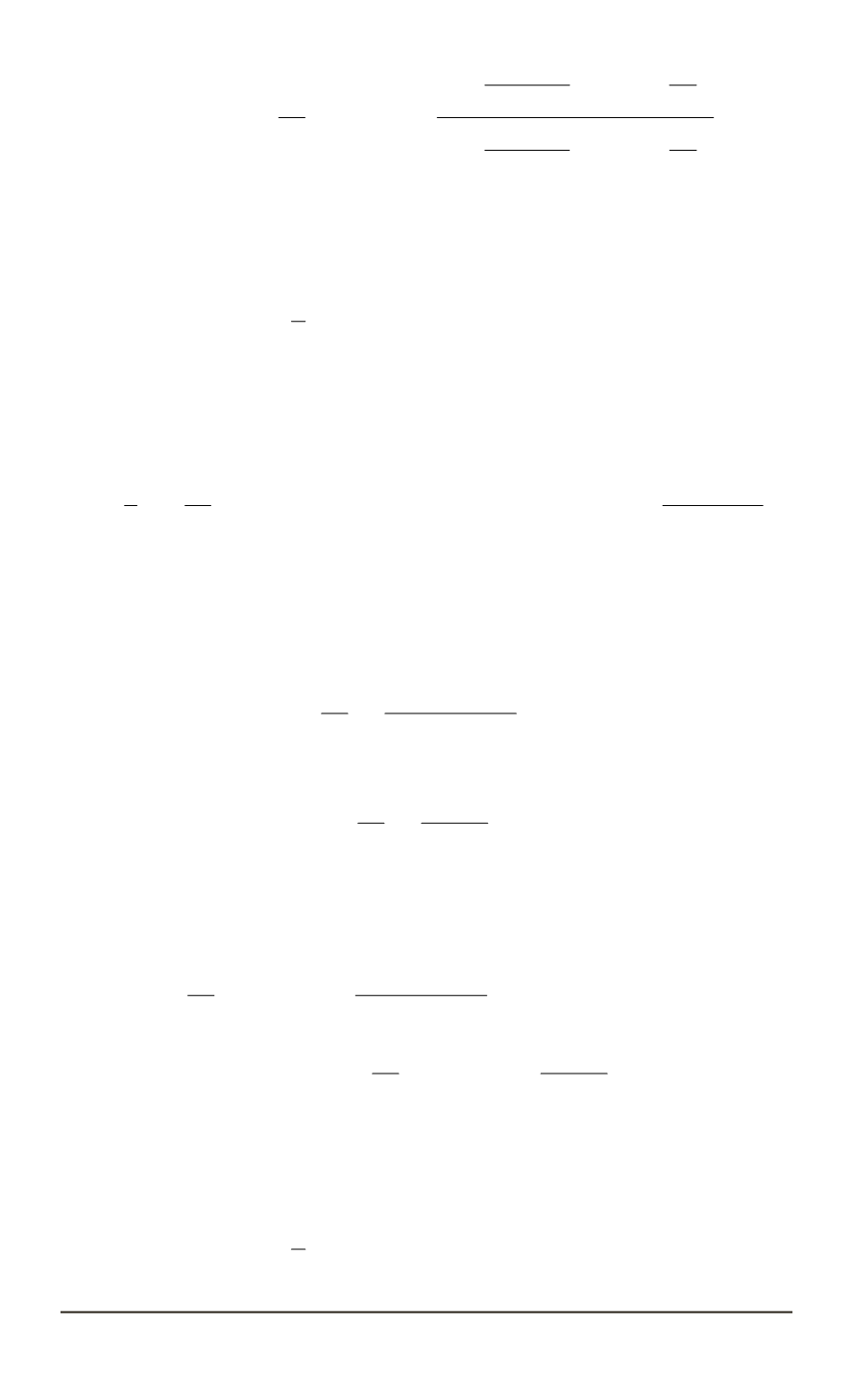
+
1
2
π
∞
Z
−∞
ψ
(
t
)ln
ch
π
(
x
−
t
)
2
a
+ sin
πy
2
a
ch
π
(
x
−
t
)
2
a
−
sin
πy
2
a
dt .
The solution to this problem is known, but the one with the kernel in
the form of an infinite series [5]:
u
(
x, y
) =
∞
Z
−∞
ϕ
(
t
)
∂
τ
G
(
x, y, t, τ
)
τ
=0
dt
+
∞
Z
−∞
ψ
(
t
)
G
(
x, y, t, a
)
dt,
where
G
(
x, y, t, π
) =
=
1
a
∞
X
0
1
μ
n
exp (
−
μ
n
|
x
−
t
|
) sin(
μ
n
y
) sin(
μ
n
τ
)
, μ
n
=
π
(2
n
+ 1)
2
a
.
Solution to the mixed boundary value problem for an infinite
layer in three-dimensional space.
For three variables kernels can not
be expressed in terms of elementary functions
:
K
2
(
x, y
) =
1
2
π
∞
Z
0
ch(
ρ
(
a
−
y
))
ch(
aρ
)
ρJ
0
(
ρ
|
x
|
)
dρ
;
L
2
(
x, y
) =
1
2
π
∞
Z
0
sh(
ρy
)
ch(
aρ
)
J
0
(
ρ
|
x
|
)
dρ.
If the functions
ϕ
(
x
)
and
ψ
(
x
)
are the functions of polynomial growth,
then the solution of the mixed problem is written as the integral formula
u
(
x, y
) =
1
2
π
Z
R
2
ϕ
(
t
)
dt
∞
Z
0
ch(
ρ
(
a
−
y
))
ch(
aρ
)
ρJ
0
(
ρ
|
x
−
t
|
)
dρ
+
+
1
2
π
Z
R
2
ψ
(
t
)
dt
∞
Z
0
sh(
ρy
)
ch(
aρ
)
J
0
(
ρ
|
x
−
t
|
)
dρ.
In this case the solution to the problem is also known with the kernel
in the form of an infinite series [5]:
u
(
x, y
) =
Z
R
2
ϕ
(
t
)
∂
τ
G
(
x, y, t, τ
)
τ
=0
dt
+
Z
R
2
ψ
(
t
)
G
(
x, y, t, a
)
dt,
8
ISSN 1812-3368. Herald of the BMSTU. Series “Natural Sciences”. 2015. No. 1

















