
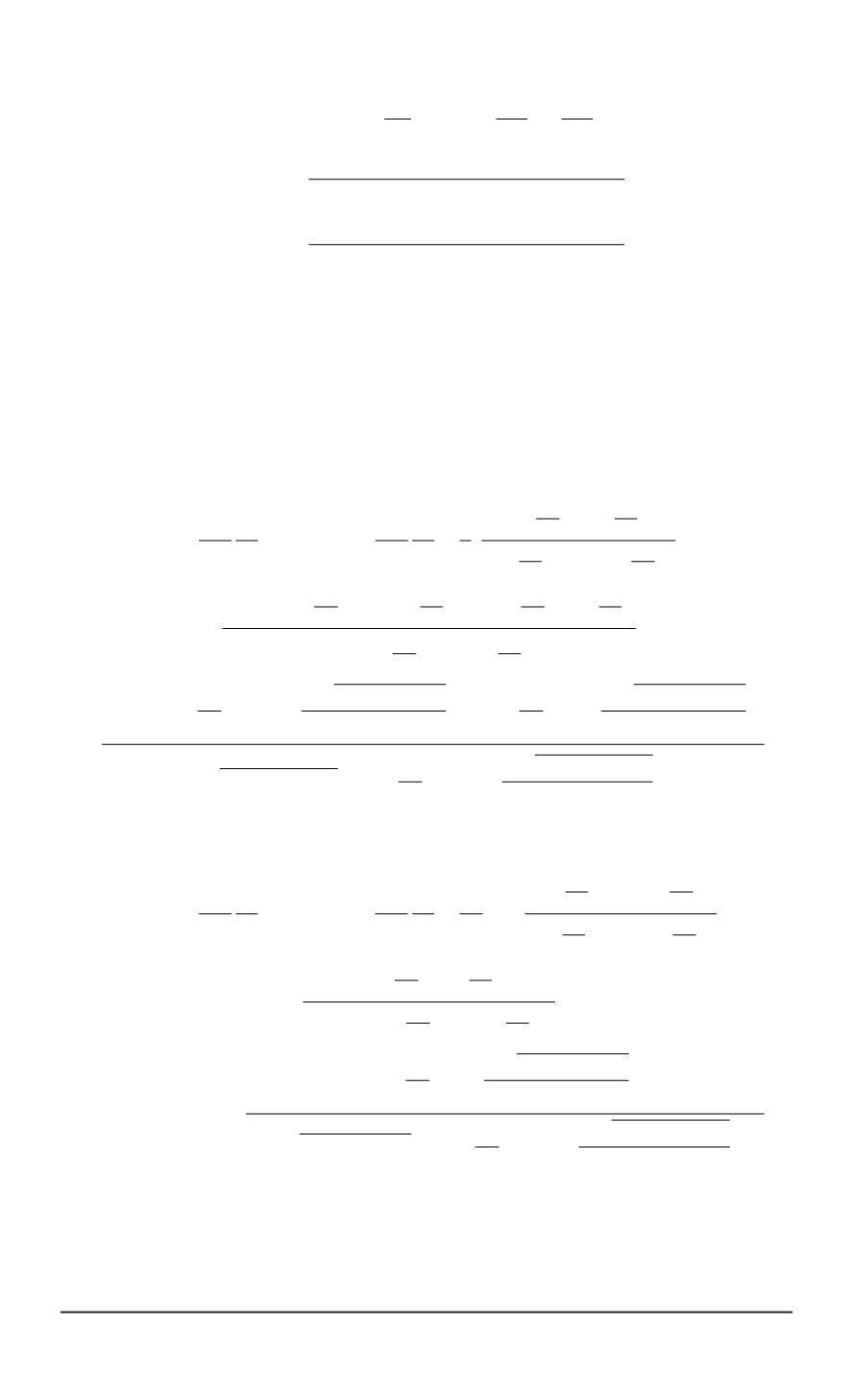
where
G
(
x, y, t, τ
) =
1
4
π
∞
X
n
=
−∞
1
r
n
1
−
1
r
n
2
;
r
n
1
=
q
|
x
−
t
|
2
+ [
y
−
(
−
1)
n
τ
−
2
na
]
2
;
r
n
2
=
q
|
x
−
t
|
2
+ [
y
+ (
−
1)
n
τ
−
2
na
]
2
.
Solution to the mixed boundary value problem for an infinite layer
in four-dimensional space.
Let us show how to apply the recurrence
relation for the solution to the mixed boundary value problem for spaces
of arbitrary dimension illustrated by the example of an infinite layer in the
four-dimensional space. We find kernels from the recurrent formulae.
K
3
(
x, y
) =
−
1
2
πr
∂
∂r
K
1
(
r, y
) =
−
1
2
πr
∂
∂r
1
a
sin
πy
2
a
ch
πr
2
a
ch
πr
a
−
cos
πy
a
=
=
2 + cos
πy
a
+ ch
πr
a
sin
πy
2
a
sh
πr
2
a
4
a
2
r
cos
πy
a
−
ch
πr
a
2
=
=
2 + cos
πy
a
+ ch
π
p
x
2
1
+
x
2
2
+
x
2
3
a
!!
sin
πy
2
a
sh
π
p
x
2
1
+
x
2
2
+
x
2
3
2
a
!
4
a
2
p
x
2
1
+
x
2
2
+
x
2
3
cos
πy
a
−
ch
π
p
x
2
1
+
x
2
2
+
x
2
3
a
!!
2
;
L
3
(
x, y
) =
−
1
2
πr
∂
∂r
L
1
(
r, y
) =
−
1
2
πr
∂
∂r
1
2
π
ln
ch
πr
2
a
+ sin
πy
2
a
ch
πr
2
a
−
sin
πy
2
a
=
=
sin
πy
2
a
sh
πr
2
a
2
πar
cos
πy
a
+ ch
πr
a
=
=
sin
πy
2
a
sh
π
p
x
2
1
+
x
2
2
+
x
2
3
2
a
!
2
πa
p
x
2
1
+
x
2
2
+
x
2
3
cos
πy
a
+ ch
π
p
x
2
1
+
x
2
2
+
x
2
3
a
!!
.
If the functions
ϕ
(
x
)
and
ψ
(
x
)
are normal functions of polynomial
growth, the solution to the mixed problem is written as the integral formula
ISSN 1812-3368. Herald of the BMSTU. Series “Natural Sciences”. 2015. No. 1
9

















