Using the substitution
x
→ −
x
we can describe the asymptotic
behavior of non-typical solutions near the right boundaries of their domains.
Combining these results we obtain the following theorem.
Theorem 2.
Suppose
k >
1
and
p
0
>
0
.
Then all maximally extended
solutions to equation
(1)
are divided into the following four types according
to their asymptotic behavior
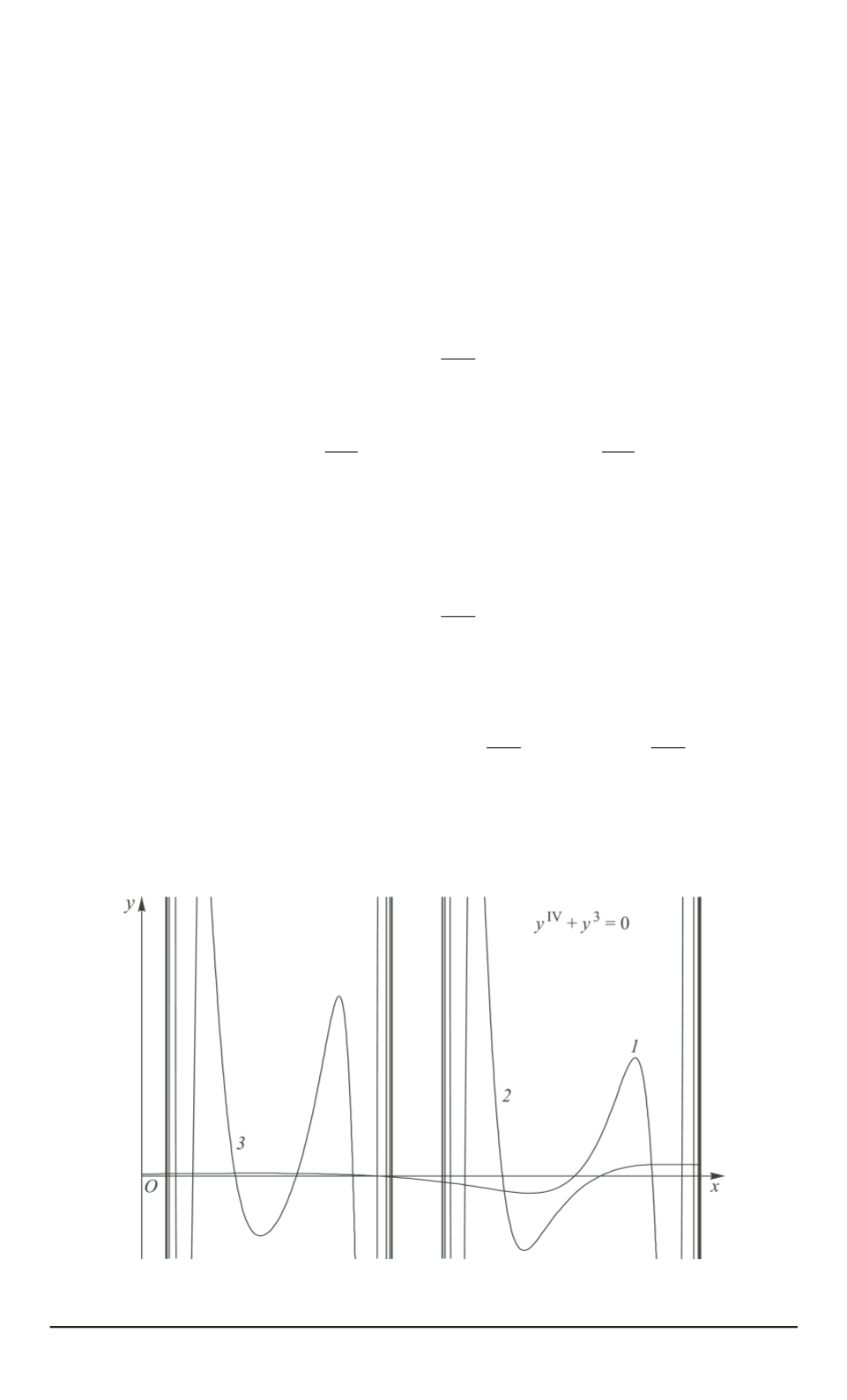
(Fig. 3)
.
0. The trivial solution
y
(
x
)
≡
0
.
1. Oscillatory solutions defined on
(
−∞
, b
)
.
The distance between their
neighboring zeros infinitely increases near the left boundary of the domain
and tends to zero near the right one. The solutions and their derivatives
satisfy the relations
lim
x
→−∞
y
(
j
)
(
x
) = 0
,
lim
x
→
b
y
(
j
)
(
x
) =
∞
for
j
= 0
,
1
,
2
,
3
.
At the points of local extremum the following estimates hold:
C
1
|
x
−
b
|
−
4
k
−
1
≤ |
y
(
x
)
| ≤
C
2
|
x
−
b
|
−
4
k
−
1
(17)
with the positive constants
C
1
and
C
2
depending only on
k
and
p
0
.
2. Oscillatory solutions defined on
(
b,
+
∞
)
.
The distance between their
neighboring zeros tends to zero near the left boundary of the domain and
infinitely increases near the right one. The solutions and their derivatives
satisfy the relations
lim
x
→
+
∞
y
(
j
)
(
x
) = 0
,
lim
x
→
b
y
(
j
)
(
x
) =
∞
for
j
= 0
,
1
,
2
,
3
.
At the points of local extremum estimates (17) hold with the positive
constants
C
1
and
C
2
depending only on
k
and
p
0
.
3. Oscillatory solutions, defined on bounded intervals
(
b
0
, b
00
)
.
All their
derivatives
y
(
j
)
,
with
j
= 0
,
1
,
2
,
3
,
4
satisfy
lim
x
→
b
0
y
(
j
)
(
x
) = lim
x
→
b
00
y
(
j
)
(
x
) =
=
∞
.
At the points of local extremum sufficiently close to any boundary
of the domain, estimates (17) hold respectively with
b
=
b
0
or
b
=
b
00
and
the positive constants
C
1
and
C
2
depending only on
k
and
p
0
.
Fig. 3. Solutions to equation (1)
ISSN 1812-3368. Вестник МГТУ им. Н.Э. Баумана. Сер. “Естественные науки”. 2015. № 2
19