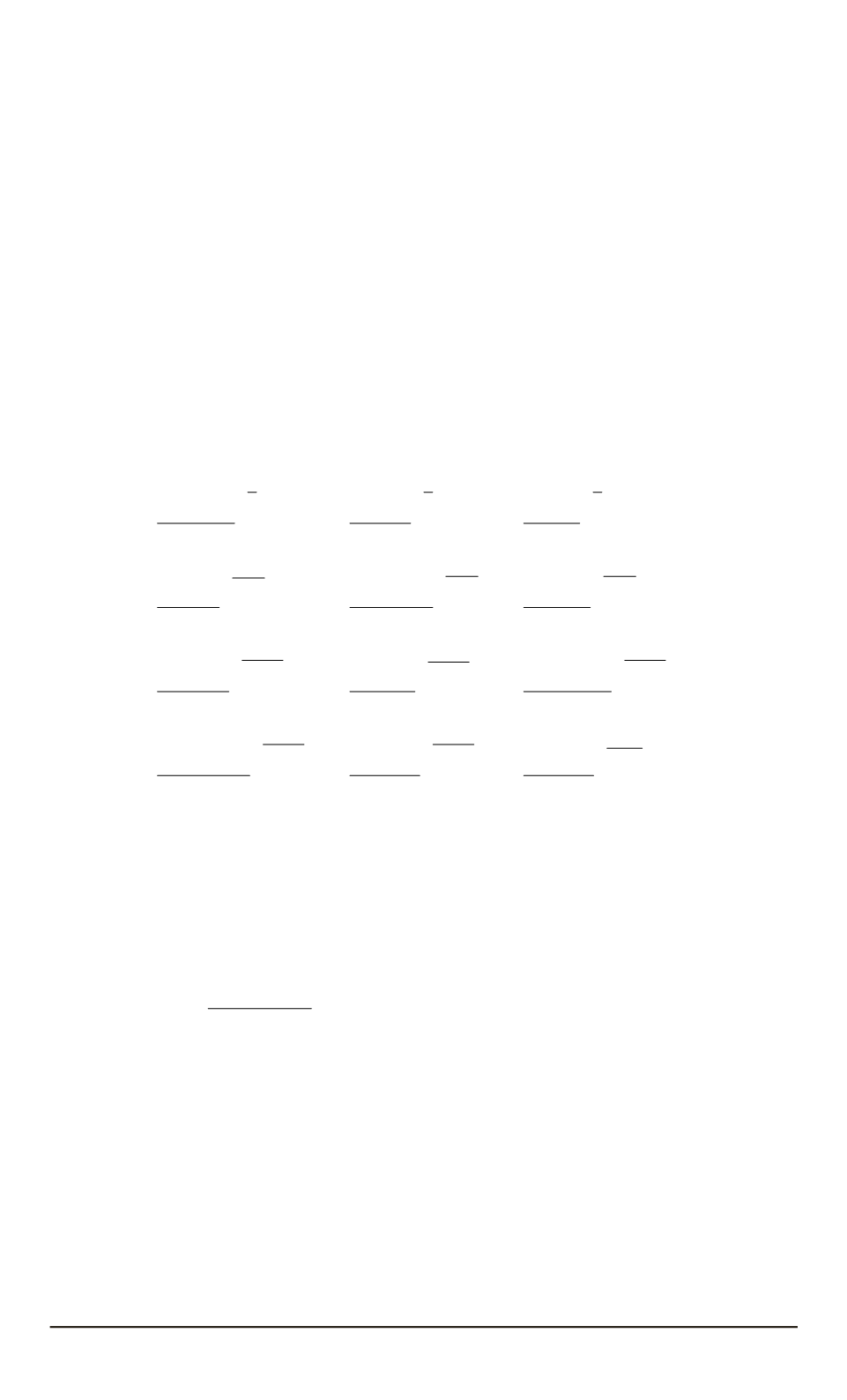
U
⊂
Ω
+
such that the above inequalities are satisfied for all solutions to
(13) with initial data
a
0
∈
U.
Hence,
|
ξ
i
(
a
0
)
−
x
i
|
< ε.
Continuity of
ξ
i
at
a
∈
Ω
+
with
a
i
>
0
is proved.
In the same way it is proved at
a
∈
Ω
+
with
a
i
<
0
.
Since
a
i
6
= 0
if
a
∈
K
j
, i
6
=
j,
we have proved continuity of the restriction
ξ
i
|
K
j
in the
case
i
6
=
j.
As for
ξ
i
|
K
i
,
note that between two zeros of
y
(
i
)
(
x
)
there exists a zero
x
j
of another derivative
y
(
j
)
(
x
)
.
The values
y
(
m
)
(
x
j
)
, m
= 0
,
1
,
2
,
3
,
due to
continuity of
ξ
j
|
K
i
,
depend continuously on
a
∈
K
i
,
whereas the restriction
ξ
i
|
K
j
depends continuously on these values. This proves continuity of the
restriction
ξ
i
|
K
i
.
I
Lemma 8.
For any
k >
1
there exist
Q > q >
1
such that for any
typical solution
y
(
x
)
to equation
(1)
the values of all expressions
y
(
x
000
j
+1
)
y
(
x
0
j
)
1
4
,
y
(
x
00
j
)
y
(
x
000
j
)
1
4
,
y
(
x
0
j
)
y
(
x
00
j
)
1
4
,
y
0
(
x
j
)
y
0
(
x
00
j
)
1
k
+3
,
y
0
(
x
000
j
+1
)
y
0
(
x
j
)
1
k
+3
,
y
0
(
x
00
j
)
y
0
(
x
000
j
)
1
k
+3
,
y
00
(
x
0
j
)
y
00
(
x
000
j
)
1
2
k
+2
,
y
00
(
x
j
)
y
00
(
x
0
j
)
1
2
k
+2
,
y
00
(
x
000
j
+1
)
y
00
(
x
j
)
1
2
k
+2
,
y
000
(
x
00
j
+1
)
y
000
(
x
j
)
1
3
k
+1
,
y
000
(
x
0
j
)
y
000
(
x
j
)
1
3
k
+1
,
y
000
(
x
j
)
y
000
(
x
0
j
)
1
3
k
+1
with sufficiently large
j
are contained in the segment
[
q, Q
]
.
J
Let us define the continuous functions
ψ
ijl
:
K
i
→
R
(all indices
i, j, l
are from 0 to 3 and pairwise different) taking each point
a
∈
K
i
to the ratio of the absolute values of the
j
-th derivative of the solution
y
(
x
)
to (13) at 0 and at the next point where the
l
-th derivative vanishes,
i.e.
ψ
ijl
(
a
) =
a
j
y
(
j
)
(
ξ
l
(
a
))
(both the numerator and the denominator are
non-zero if
a
∈
K
i
).
Due to Lemma 6, each function
ψ
ijl
at all points of the compact set
K
i
is positive and less than 1. Hence
0
<
inf
K
i
ψ
ijl
(
a
)
≤
sup
K
i
ψ
ijl
(
a
)
<
1
.
Now consider an arbitrary typical solution
y
(
x
)
to (1) and two its nodes,
say
x
0
j
and
x
000
j
+1
,
with sufficiently large numbers such that the related points
in
S
3
belong to
Ω
1
+
.
In this case we can choose constants
A
6
= 0
and
B >
0
such that the function
z
(
x
) =
Ay
(
Bx
+
x
0
j
)
is a solution to (13) with
a
∈
K
1
.
Indeed, this is equivalent to existence of
A
6
= 0
and
B >
0
such
ISSN 1812-3368. Вестник МГТУ им. Н.Э. Баумана. Сер. “Естественные науки”. 2015. № 2
15